Assignment 7

by

Jackson Huckaby

For this assignment, we will explore how to construct a circle that is tangent to two other given circles and with one point given.

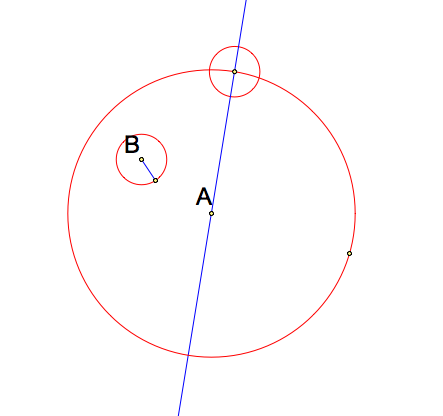
To begin we will construct a line through the center of circle A. At the intersection of the this line and the larger circle we construct a smaller circle with the same radius as circle B.

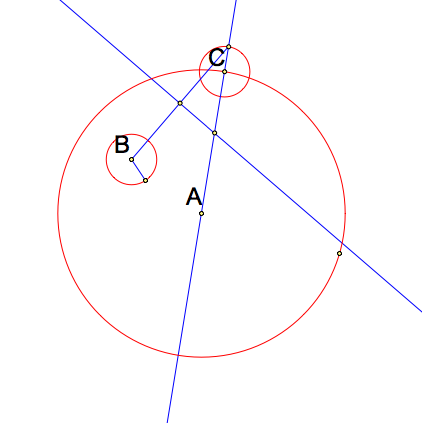
Next we will construct a length between the intersection of our new smaller circle and the line through circle A and the center of circle B. We then construct the perpendicular bisector of this new line.

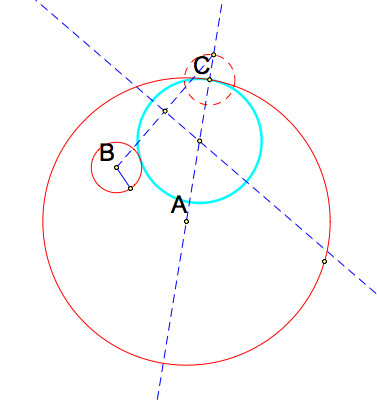
What we have achieved by doing this is we have located the center point of the circle that is tangent to both circle A and B. This center is located at the intersection of our perpendicular line and our original line through circle A.

A script tool for this construction can be found here.

Now we want to consider what the locus of all such tangent circles will be, to do so I have traced the intersection in yellow on GSP.

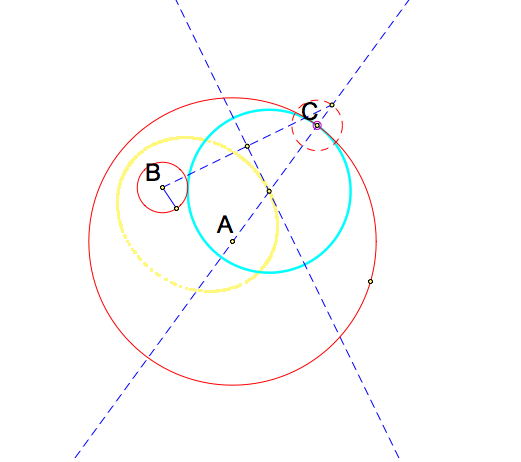
What we seem to have is the drawing of an ellipse in our circles, with the focal points of this ellipse being the centers of circle A and circle B.

Try to prove this.

To create this tangent circle we ask ourselves what do we need to change?

If we start from this point in our construction we can alter our tangent circle by simply constructing our next segment from the inside intersection point of circle C and the line through circle A instead of the outer intersection.

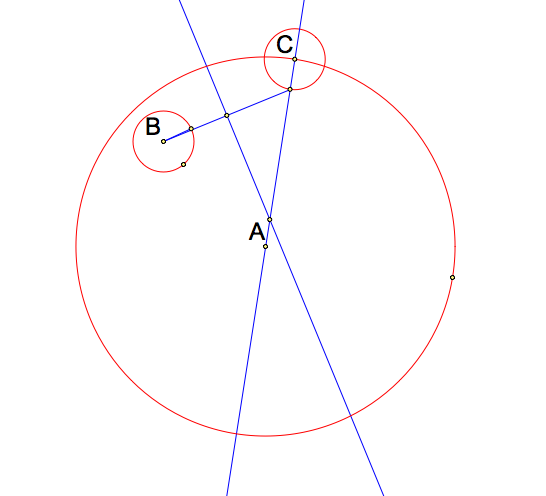
And then by finding the midpoint again we get our new desired tangent circle.

So is the locus of this tangent circle still our ellipse? Thoughts?

Lets trace it and see.

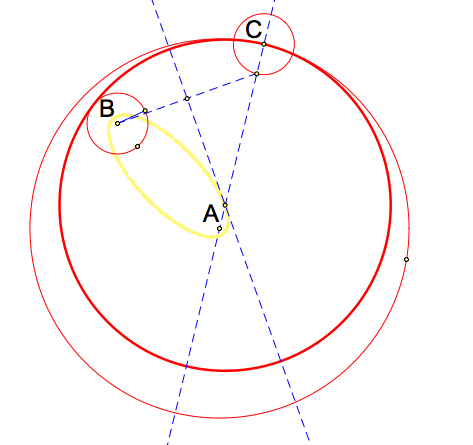
It is!

Here is a script tool for this circle.

So what would happen if our two given circles intersected?

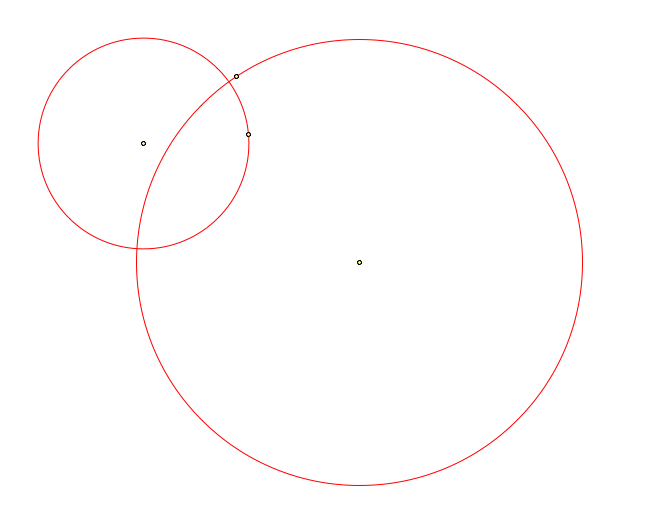
Instead of doing our constructions again, we can use the script tools just created to quickly construct both tangent circles.

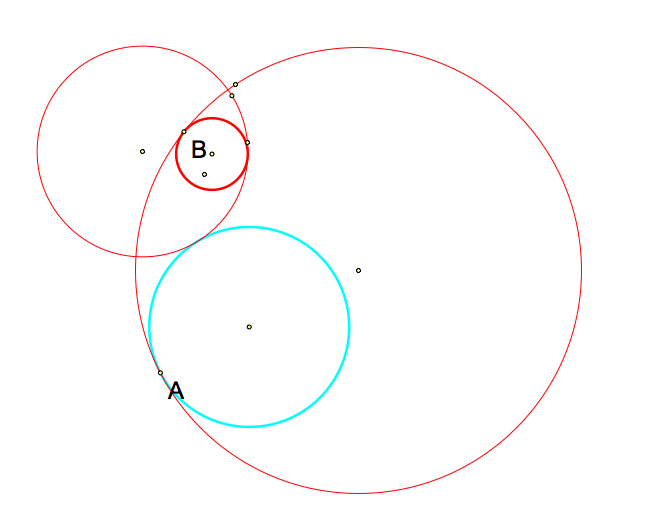
Next we will construct the locus of both centers of the tangent circles.

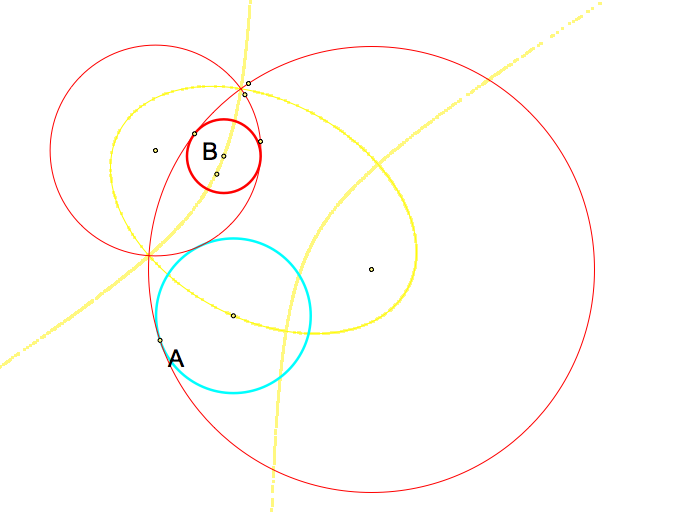
What we see is the Locus of the blue circle gives us an ellipse like before, however the locus of the red circle gives us a hyperbola.

A script tool can be found here.

If the two original circles are outside of each other, like so:

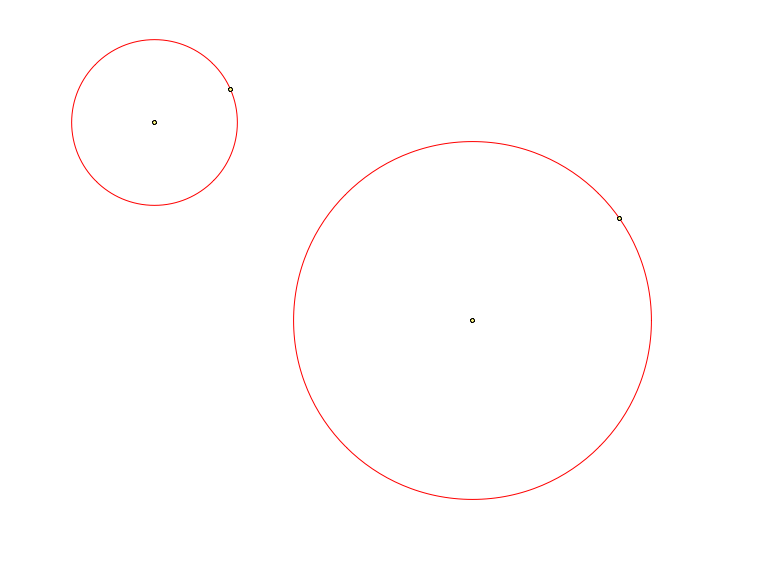
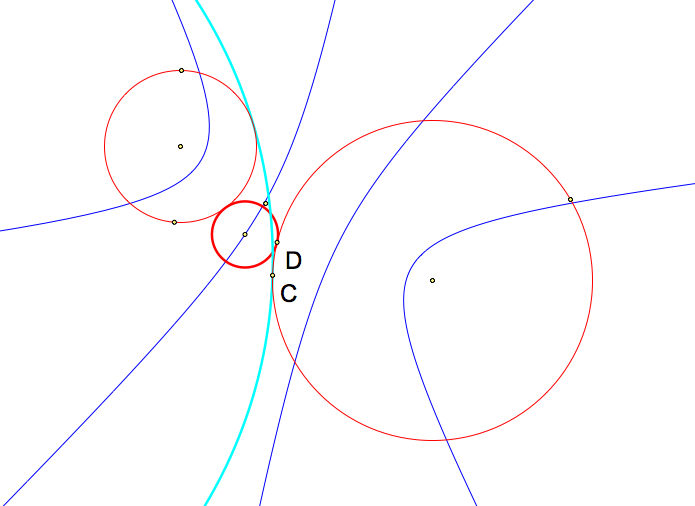
Then both of our loci become hyperbolas.

Return home here.